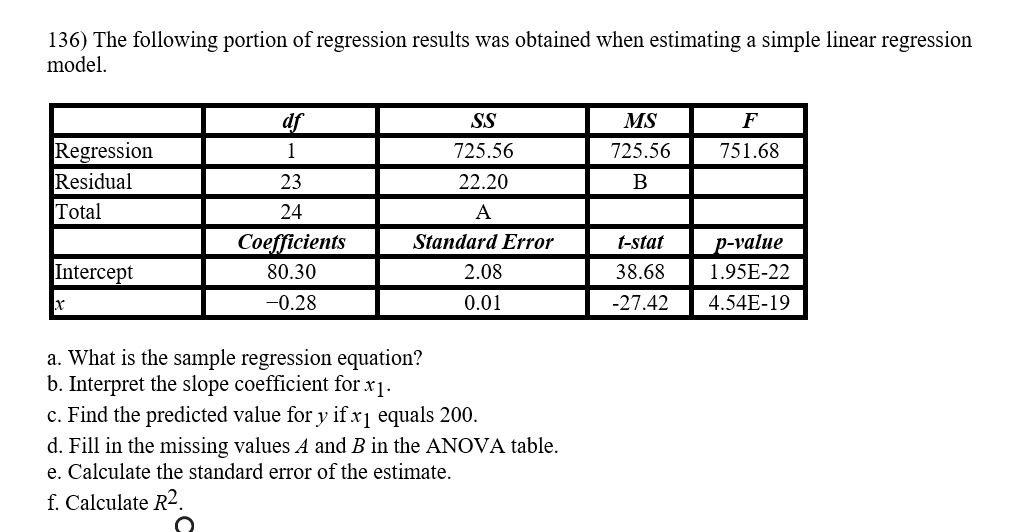
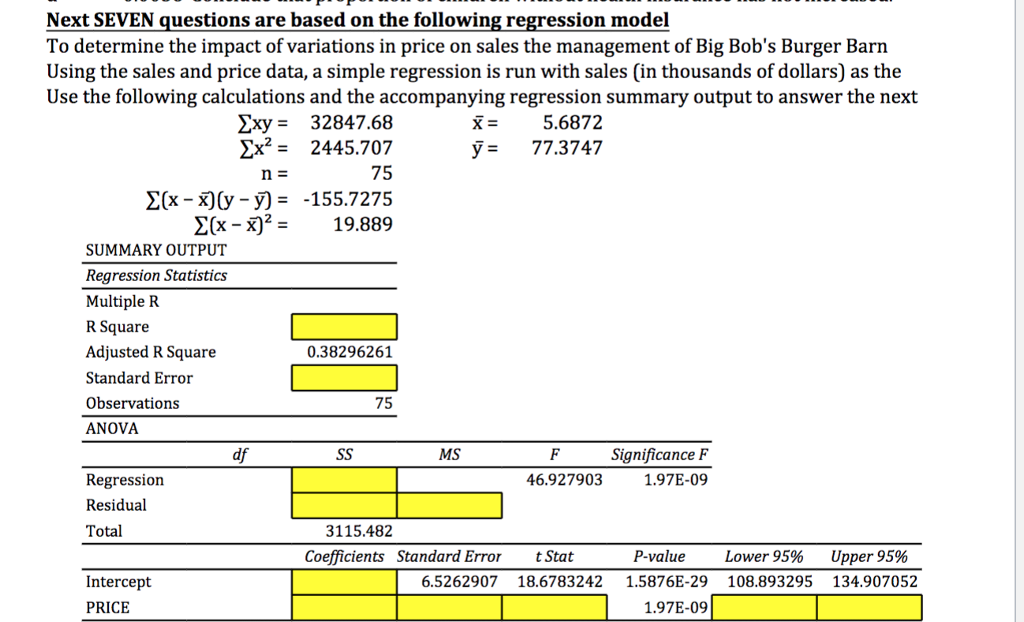
hooglfox.blogg.se - Calculate standard error of slope

Calculate standard error of slope skin#
Calculate standard error of slope software#

Then the first two rows of output are extended to number of columnsĮquals number of regressors (including constant)Īnd the last three rows are the same as earlier. LINEST can be extended to multiple regression (more than an LINEST(A2:A6,B2:B6,1,0),Īnd then highlight cells A8:B8, say, hit F2 key, and hit To get just the coefficients give the LINEST command with the lastĠ rather than 1, ie. The estimated coefficients have standard errors of, respectively, Hit the F2 key (Then edit appears at the bottom left of the.We consider an example where output is placed in the array D2:E6.įirst in cell D2 enter the function LINEST(A2:A6,B2:B6,1,1). (as here there are two regressors), so we need to use an array formula. The formula leads to output in an array (with five rows and two columns Several forecasts from a two-variable regression. The individual function LINEST can be used to get regression With R-squared of 0.8 and estimated standard deviation of u of 0.36515Īnd we forecast that for x = 6 we have y = 0.8 + 0.4*6 = 3.2. FORECAST(6,A1:A6,B1:B6) yields the OLS forecast value ofįor X=6 (forecast 3.2 cars for household of size 6).STEYX(A1:A6,B1:B6) yields the standard error of the regression.RSQ(A1:A6,B1:B6) yields the R-squared of 0.8.SLOPE(A1:A6,B1:B6) yields the OLS slope estimate of 0.4.INTERCEPT(A1:A6,B1:B6) yields the OLS intercept estimate.The individual functions INTERCEPT, SLOPE, RSQ, STEYX andįORECAST can be used to get key results for two-variable We wish to estimate the regression line: y = The population regression model is: y = β 1

REGRESSION USING EXCEL FUNCTIONS INTERCEPT, SLOPE, RSQ, STEYX and It is easier to instead use the Data Analysis Add-in for Regression.

Fitting a regression line using Excel function LINEST.įor most purposes these Excel functions are unnecessary.

Fitting a regression line using Excel functions INTERCEPT, SLOPE,.

This January 2009 help sheet gives information on Again, the P-value is the answer to the question "how likely is it that we’d get a test statistic t* as extreme as we did if the null hypothesis were true?" The P-value is determined by referring to a t-distribution with n-2 degrees of freedom.EXCEL 2007: Two-Variable Regression using function LINEST EXCEL 2007: Two-Variable Regression using function LINEST A. Third, we use the resulting test statistic to calculate the P-value. Second, we calculate the value of the test statistic using the following formula: However, we can test values other than 0 and the alternative hypothesis can also state that β 1 is less than () some number β.

Calculate standard error of slope software#

By default, statistical software conducts the hypothesis test with null hypothesis, β 1 is equal to 0, and alternative hypothesis, β 1 is not equal to 0. Most often, however, we are interested in testing whether β 1 is 0. The phrase "some number β" means that you can test whether or not the population slope takes on any value. Null hypothesis H 0 : β 1 = some number βĪlternative hypothesis H A : β 1 ≠ some number β First, we specify the null and alternative hypotheses: We follow standard hypothesis test procedures in conducting a hypothesis test for the slope β 1. An α-level hypothesis test for the slope parameter β 1 That is, we want to know if the population slope β 1 is unlikely to be 0.

Calculate standard error of slope skin#

But, we want to know if there is a relationship between the population of all of the latitudes and skin cancer mortality rates. Is there a relationship between state latitude and skin cancer mortality? Certainly, since the estimated slope of the line, b 1, is -5.98, not 0, there is a relationship between state latitude and skin cancer mortality in the sample of 49 data points. And a plot of the data with the estimated regression equation looks like: